The Birthday Problem

What is the probability that in a group of 4 people, at least two are born in the same month?
You may assume a 365 day year and that all months have the same probability (which they do not).

You solve this the same way that you solve the original Birthday Problem.
First, determine the probability that all four people are born in different months:
The first person could be born in any of the 12 months: (12/12)
The second person could be born in any of the 11 months remaining: (11/12)
The third person could be born in any of the 10 remaining months: (10/12)
The fourth person could be born in any of the 9 remaining months: (9/12)
So the probability that all four are born in different months is:

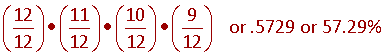
Therefore the probability that at least 2 of them are born in the same month is

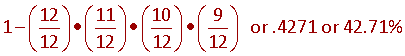
But this is assuming that the probabilities for the months are equal, but in fact, they are not. Seven months have 31 days, four have 30 days, and one month has 28 days. So, let's do this problem right!

What is the probability that in a group of 4 people, at least two are born in the same month?
You may assume a 365 day year, but this time, let's use the correct probabilities for the months.

For each of the seven months containing 31 days, the probability of being born in that month is 31/365.
For each of the four months containing 30 days, the probability of being born in that month is 30/365.
For February which contains 28 days, the probability of being born in that month is 28/365.

Now, we solve this problem in a similar manner to the one above. We must figure out the probability that all four are born in different months, and then subtract that number from 1 to get the probability that at least two are born in the same month.

We must first determine the number of combinations of four DIFFERENT months. Using ₁₂C₄, we get 495 different combinations of 12 months taken 4 at a tiime. Luckily, we can break this down into nine categories since many of those 495 combinations would have the same probability:
JAN-FEB-MAR-APR would have the same probability as JAN-FEB-SEP-DEC because they each have two months with 31 days, 1 month with 30 days, and 1 month with 28 days.

So, here are the nine categories:

Category 1st Month 2nd Month 3rd Month 4th Month Number of Combinations

#1 31 31 31 31 ₇C₄ = 35

#2 30 30 30 30 ₄C₄ = 1

#3 31 31 31 30 ₇C₃ x 4 = 140

#4 31 31 31 28 ₇C₃ x 1 = 35

#5 31 31 30 30 ₇C₂ x ₄C₂ = 21 x 6 = 126

#6 31 31 30 28 ₇C₂ x 4 x 1 = 21 x 4 x 1 = 84

#7 31 30 30 30 7 x ₄C₃ = 7 x 4 = 28

#8 31 30 30 28 7 x ₄C₂ x 1 = 42

#9 30 30 30 28 ₄C₃ x 1 = 4

Total 495

Now the next table shows the probabilty that all four people have different birth months for each of the nine categories:

Category 1st Month 2nd Month 3rd Month 4th Month Probability that all are different

#1 31 31 31 31 (31/365)⁴ = .00005203

#2 30 30 30 30 (30/365)⁴ = .00004564

#3 31 31 31 30 (31/365)³ x 30/365 = .00005036

#4 31 31 31 28 (31/365)³ x 28/365 = .00004700

#5 31 31 30 30 (31/365)² x (30/365)² = .00004873

#6 31 31 30 28 (31/365)² x 30/365 x 28/365 = .00004548

#7 31 30 30 30 31/365 x (30/365)³ = .00004716

#8 31 30 30 28 31/365 x (30/365)² x 28/365 = .00004014

#9 30 30 30 28 (30/365)³ x 28/365 = .00004259

Now, we must combine the two tables to obtain the sum of all 495 combinations:
Sum = 35 x .00005203 + 1 x .00004564 + 140 x .00005036 +
35 x .00004700 + 126 x .00004873 + 84 x .00004548 +
28 x .00004716 + 42 x .00004014 + 4 x .00004259
= .02369911

However, this only represents the nine combinations. We have computed the probabilities for each combination of four months. But we have ignored the possibility of the four people having different birth months within the same category.
For example:

Abe could be born in JAN, Ben in FEB, Carol in MAR, and David in APR; or
Abe could be born in JAN, Ben in FEB, Carol in APR, and David in MAR; or
Abe could be born in JAN, Ben in MAR, Carol in FEB, and David in APR; or
Abe could be born in JAN, Ben in MAR, Carol in APR, and David in FEB; or

Abe could be born in JAN, Ben in APR, Carol in FEB, and David in MAR; or
Abe could be born in JAN, Ben in APR, Carol in MAR, and David in FEB; or
Abe could be born in FEB, Ben in JAN, Carol in MAR, and David in APR; or
Abe could be born in FEB, Ben in JAN, Carol in APR, and David in MAR; or

Abe could be born in FEB, Ben in MAR, Carol in JAN, and David in APR; or
Abe could be born in FEB, Ben in MAR, Carol in APR, and David in JAN; or
Abe could be born in FEB, Ben in APR, Carol in MAR, and David in JAN; or
Abe could be born in FEB, Ben in APR, Carol in JAN, and David in APR; or

Abe could be born in MAR, Ben in JAN, Carol in FEB, and David in APR; or
Abe could be born in MAR, Ben in JAN, Carol in APR, and David in FEB; or
Abe could be born in MAR, Ben in FEB, Carol in JAN, and David in APR; or
Abe could be born in MAR, Ben in FEB, Carol in APR, and David in JAN; or

Abe could be born in MAR, Ben in APR, Carol in JAN, and David in FEB; or
Abe could be born in MAR, Ben in APR, Carol in FEB, and David in JAN; or
Abe could be born in APR, Ben in JAN, Carol in FEB, and David in MAR; or
Abe could be born in APR, Ben in JAN, Carol in MAR, and David in FEB; or

Abe could be born in APR, Ben in FEB, Carol in MAR, and David in JAN; or
Abe could be born in APR, Ben in FEB, Carol in JAN, and David in MAR; or
Abe could be born in APR, Ben in MAR, Carol in JAN, and David in FEB; or
Abe could be born in APR, Ben in MAR, Carol in FEB, and David in JAN; or

So, for each category of four months, there are 24 permutations!
So, we must multiply our probability sum by 24 to get 24 x .02369911 = .56877864.
This means that the probability that four people will have different birth months is .56877864.

And finally, the probability that at least two of the four are born in
the same month is 1 - .56877864 = .43122136 or 43.12%.

What I find interesting is that it is very close to the probability above! Assuming all months have the same probability, we got 42.71%.